The Sato-Tate Conjecture

Elliptic Curves

The original Sato-Tate conjecture wasn't about modular forms, but about a class of geometric objects called elliptic curves. These curves are defined by equations similar to the following: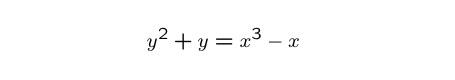

For any prime number p let N(p) be the number of integral solutions (x,y) to this equation that are different modulo p.

p	N(p)	a_p	\|a_p\|p^-1/2
2	2	0	0
3	3	0	0
5	7	-2	0.89
7	7	0	0
11	11	0	0
13	7	6	1.66
17	15	2	0.49
19	19	0	0
23	23	0	0
29	39	-10	1.86
31	31	0	0
37	39	-2	0.33

In addition to N(p) we consider the numbers a_p defined by

By a theorem of Hasse from the 1930's,

for almost all primes p. Consequently, we can write

with a Frobenius angle φ_p between 0 and π. Sato and Tate conjectured around 1960 that these angles satisfy the sin² law, that is, they are distributed according to the function

The following graphs give some evidence for this conjecture.

Sato-Tate Graphs for the Elliptic Curve y²+y=x³-x (1000 Parameters, Step 1)

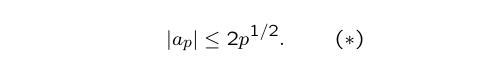
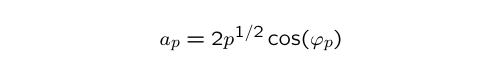
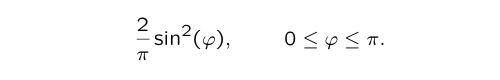
By the celebrated Modularity Theorem, the Sato-Tate conjecture for elliptic curves is actually a consequence of the Sato-Tate conjecture for modular forms. Namely, the Modularity Theorem states that the numbers a_p defined above for an elliptic curve are in fact the Fourier coefficients of a modular form (a cuspidal eigen-newform of weight 2, to be precise). Hence, if we know the sin² law for modular forms, we know it for elliptic curves.

We should remark that the modular forms in the Modularity Theorem are not exactly of the type that we defined on page 2. It is necessary to consider modular forms with ''level''. For example, the modular form corresponding to the elliptic curve y²+y=x³-x above is the essentially unique cusp form of weight 2 and level 11.

There is still more - go to page 4.


Parameters: